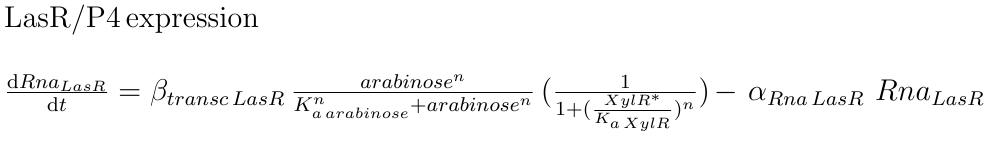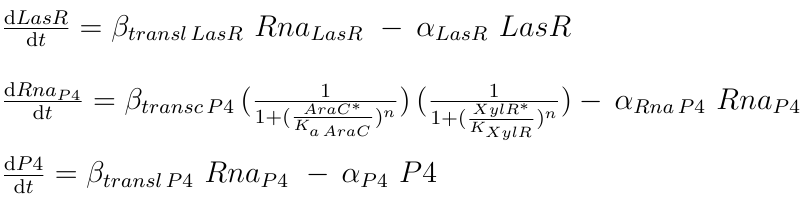Sweet AND

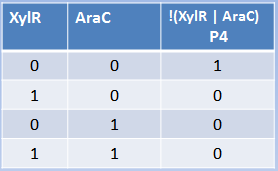
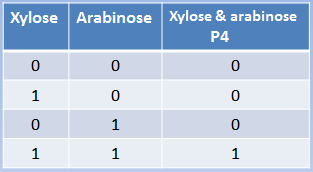
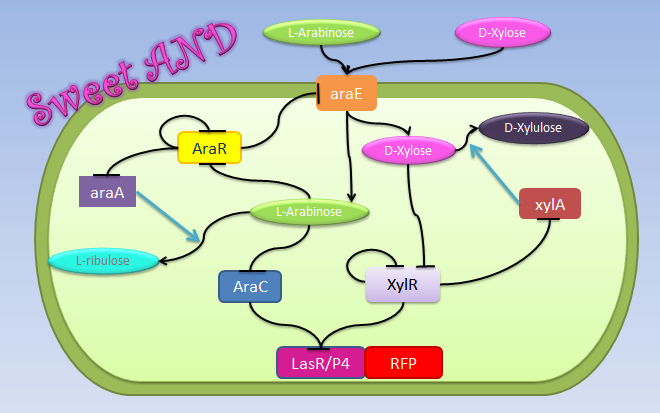
Xylose and arabinose are sugars used by bacteria as carbon sources. XylR and AraC are transcriptional regulators modulated by xylose and arabinose. In this case, the hybrid promoter has as backbone made of the pBAD promoter plus a binding site for XylR. XylR represses the transcription until xylose is present in the cell, the same way that ArsR and CzrA work. AraC represses through the formation of DNA looping. When arabinose enters the cell, it dissolves the loop and transcription starts. We can conclude that arabinose and xylose are essential for the expression of genes under the pBAD/xylR promoter, thus acting as an AND Boolean operation.

Repressors

Inputs

The behavior of both ANDs predicted by our model is consistent with what we expected. From the perspective of the repressors, a higher level of PoPs (Polymerase Per Second, which is a measurement of the level of transcription) is reached when both repressor concentrations are 0, and PoPs start to fall when the concentration of repressors increases, reaching the highest level of repression (lower PoPs) at high concentration of both repressors. This behavior reminds us of the way a NOR behaves, because at low levels of repressor PoPs is high, but for repression, high concentration of one repressor is enough. However, we can't control (directly) the concentration of repressors, so the right way to look at the system is from the perspective of the Input: the metals or the sugars.

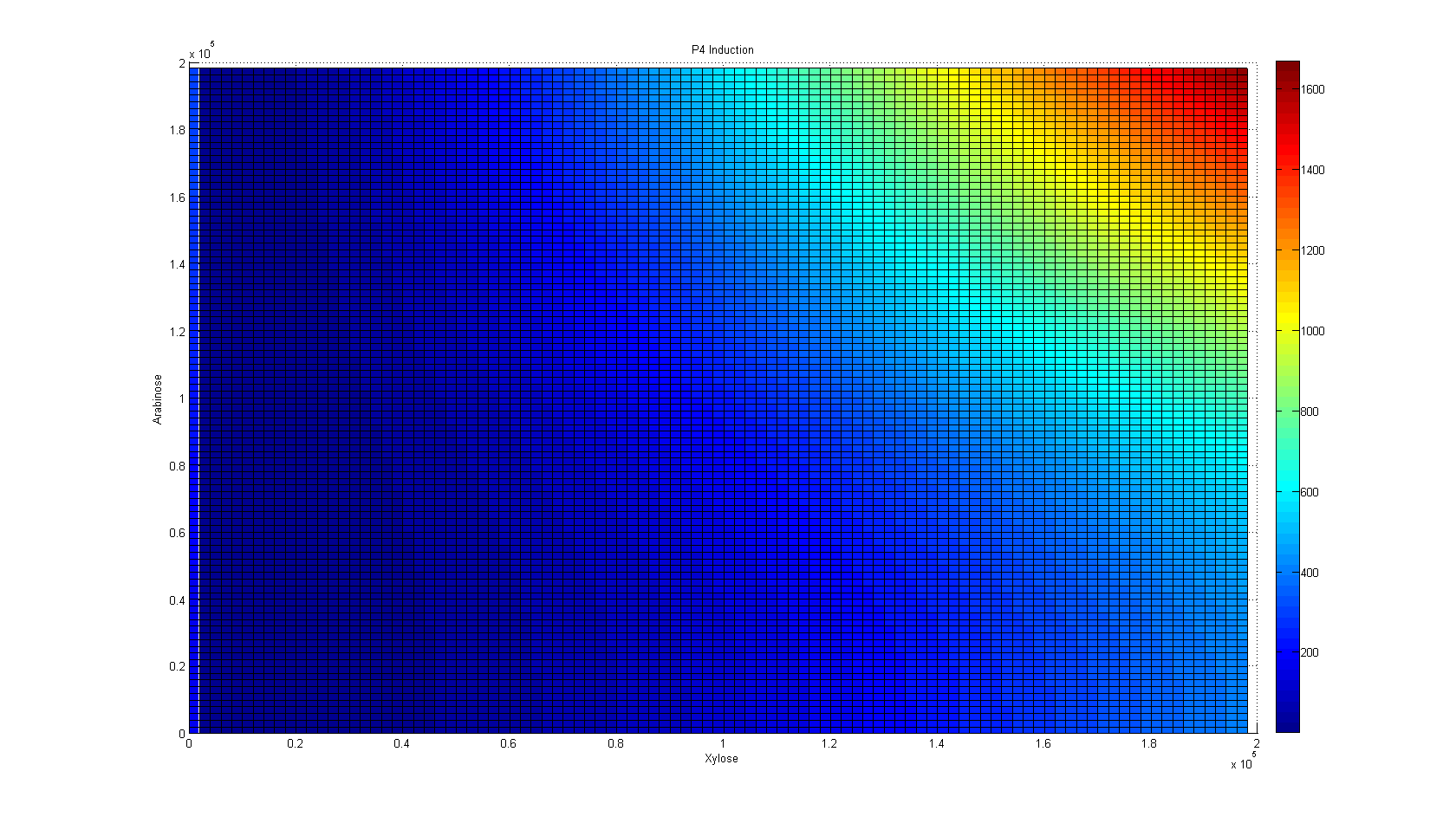
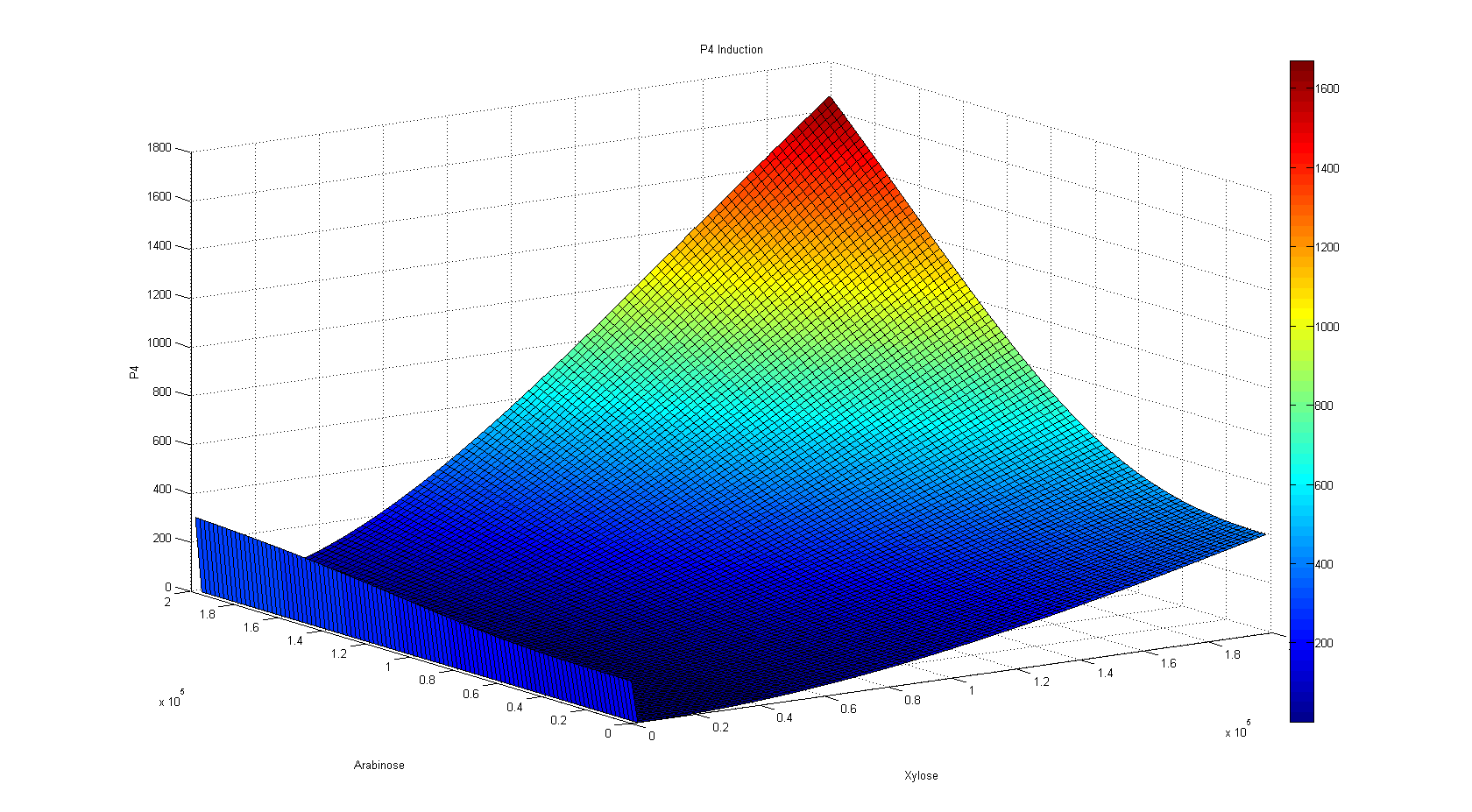
If we looked PoPs as a function of the Input, it behaves as an AND logic gate, as can be seen in the continuous TRUTH TABLE. At lower concentrations of Input, the PoPs level is in a very repressed stage. If you keep adding concentration of one of the Input on only one of the axes (yes, axes is the plural of axis), there won’t be high activation until the addition of high concentrations on the other axis. PoPs will increase as the Input concentration increases in a direct proportion fashion.

KAPPA

Sometimes people wonder why we used Kappa, a stochastic approach, to model our system, which was also described by deterministic differential equations. First of all, Kappa helped us come up with the concentration ranges entered in the differential equations (here, an exclamation of awe is usually heard). How did this come to happen? Well, the simulation in kappa is driven by the binding events and is dependent on the species concentrations, so using general kinetic rates we were able to see the dynamic behavior given by the architecture of the network. This allowed us to approximate the final species concentration and look for parameters missing in our ODE system, which was something useful, since we were as lost as a three-legged dog in a rodeo dance.

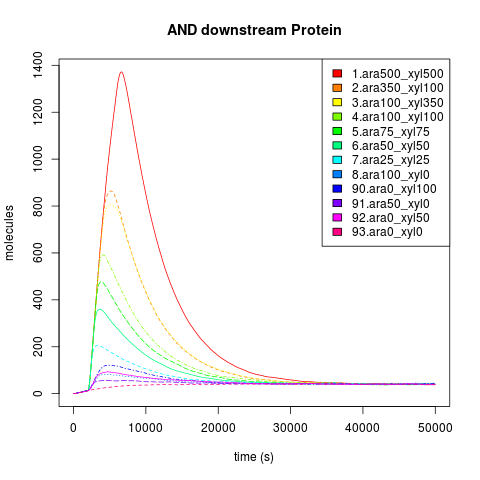
So, first we ran the and_sugar.ka kappa script shifting the sugar initial concentrations in the single input burst and getting the average of 100 iterations to eliminate the noise in the simulation. After running some simulation time, we were happy to see that our "Sweet AND" behaved as expected, that is as an AND. Besides, we were able to see the maximal concentration of the protein that would be downstream of our AND, and the time it took to reach it. As you can see, our simulation grid is not described in its entirety. This is because of the high kappa simulation time consumption, so we chose to feed our ODE system with some parameters and scan the system in a broader manner instead of dying of old age waiting for Kappa. Nevertheless, some behaviors can only be noticed by looking at the simulation grid. For example, the activation of P4 depending on the xylose and arabinose concentrations. As it becomes apparent through a meticulous and insightful analysis only doable by a really smart, handsome, and admirable person, the activation of P4 depends on the concentrations of both, xylose and arabinose. When only one of the two sugars has a high concentration, the activation is not as elevated as when the two have high concentrations.

Xylose	500,000
	350,000
	100,000
	75,000
	50,000
	25,000
	0.0
		0.0	25,000	50,000	75,000	100,000	350,000	500,000
		Arabinose

We can focus only in the dynamics of the downstream protein instead of the dynamic of all the system. This plot describes the time to reach steady state and maximal concentration of the AND downstream protein. It also describes what the maximal concentration of the AND protein is. All of this is based on a single input burst concentration.

Endogenous system and Downstream protein dynamic is dependent of the kind of input.

The inputs used by each AND have a unique effect in the response of the cell, because carbohydrates are degraded by the cell. When we add sugars to the sweet AND system, if they are added just like a discrete and unique dose of them, the cell will react to the alteration through the activation of the degradation machinery, and when the sugar pool gets empty, the cell achieves a similar state to the one before the sugar was introduced. When the sugar addition is constant, the cell has a complete phenotypic change, reaching a new steady state.

Transfer Function

Our logic AND gates are fully dependent on the intracellular concentrations of the inducer (in this case, xylose and arabinose). Then, to accomplish our duty in model, we use the "Transfer Function", for which it is necessary to take into account the regulation and dynamics of the endogenous B. subtilis intake-efflux system that controls the intracellular concentrations of sugars. All of this is going to be the "Black Box".

So, our first task was to reconstruct the regulatory network of B. subtilis for the intake-efflux system. The regulation data was retrieved from several papers and databases like [http://bsubcyc.org/ http://bsubcyc.org/]

Sweet AND gate: Arabinose and xylose are imported into the cell by the araE permease. The carbon metabolism system is highly regulated in order to optimize the consumption of sugars using the least amount of energy. XylR and AraR are repressors responsible for the regulation of genes involved in the metabolism and intake of xylose and arabinose, respectively. Although XylR is not well characterized, some studies suggest a correlation between XylR and the concentration of xylose inside the cell. AraR not only represses the production of genes like araE or araA, but it also represses itself. AraA and xylA are isomerases that convert L-arabinose into L-ribulose and D-xylose into D-xylulose. XylR from B.subtilis is also used by our construction as a repressor, but the production of xylR is the product of the endogenous production plus the production because of a constitutive promoter. Under that constitutive promoter, AraC is also produced for the regulation of the hybrid promoter. The output of the AND is the production of a transcription factor, LasR or P4, and RFP.

The suggested amount of each input that activates the gate according to our model is:

Deterministic model description

Our team made both, a deterministic and a stochastic approach. Here, we are going to describe the deterministic model. General assumptions:

●This model's scope is single cell. While the regulation steps were modeled through Hill equations, other reactions were treated under Michaelis-Menten assumptions, notably isomerizations and transport processes (i.e. those that involved energy consumption).

●The maximal transcription rate for the system species was obtained by dividing the maximum number of nucleotides processed by the RNA polymerase (i.e. maximum polymerase activity), by the length of the nucleotide sequence.

●Translational rates were obtained by getting the maximum number of amino acids added by the ribosome divided by the length of the protein in amino acids.

●The protein degradation rate is considered as equal for all the protein species in the model.

●The degradation rate for mRNAs is equal for all the different species of mRNAs.

●The different binding sites of the same transcription factor have the same affinity to the TF.

●For each mRNA species, its concentration will be the sum of the production as affected by its respective promoter and the transcription factors that regulate it, minus the degradation of the mRNA at that time.

●For each protein, the change in its concentration depends in the amount of protein produced by translation minus the degradation rate of the protein.

●The Hill coefficients tend to be 2.

●The parameters of concentration were expressed in terms of molecules per cell(Vol of a B.subtilis cell ~10^-14) and the time units were converted sec.

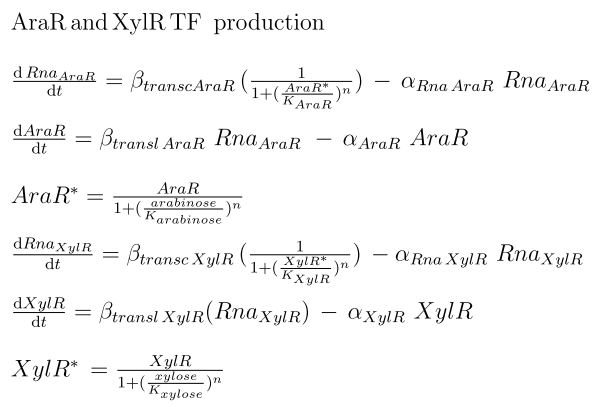
Deterministic model

Xylose and arabinose are part of the pool of molecules that can be used by B. subtilis as carbon sources. Among membrane transporters, affinity for particular kinds of sugars is often high; nevertheless AraE is the main permease responsible for the intake of arabinose, xylose, and galactose monosaccharides. AraE is down-regulated by AraR, which also represses some other genes involved in arabinose metabolism. AraR is inactivated by arabinose and by itself (Krispin O, 1998 [19]). These little details evidentiate the fact that arabinose is required for xylose cellular influx.

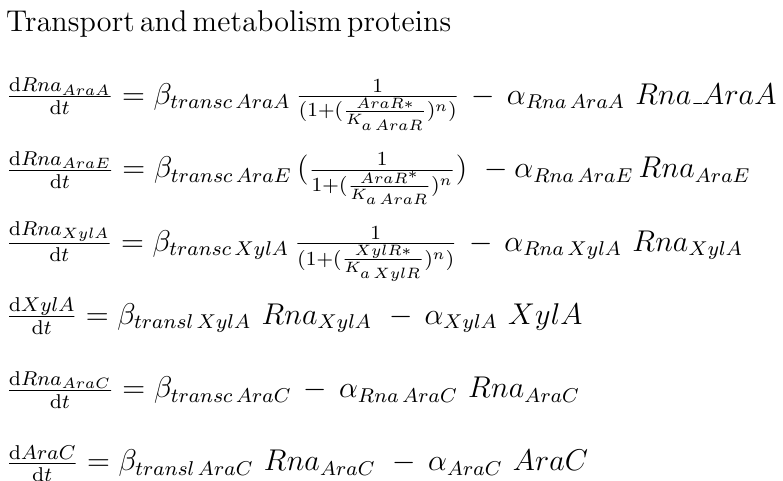
The concentrations of XylA and AraA depend on the concentration of their respective repressors, XylR and AraR, and their respective dissociation constants.

Intracellular xylose induces the production of genes involved in its metabolism, also through the inactivation of their correspondent repressor, XylR. XylA is the enzyme that catalyzes the conversion of D-xylose to D-xylulose. For arabinose, the isomerase that catalyzes the conversion of L-arabinose into L-ribulose is AraA(Gu Y, 2010 [20]).

In that way, the amount of intracellular arabinose is defined as the amount introduced by AraE minus the amount converted to L-ribulose by AraA; for xylose the process is similar but the degradation depends on xylA. We assume that the rates of intake of both sugars by AraE are similar. For the degradation rates by AraA and XylA, they are defined by Michaelis-Menten kinetics, since this kind of equation takes into account the energy consumption, and also because it is a simple reaction with one step and one substrate.

As for the amount of XylR presented in the system, we had two sources of production. One is the endogenous production in Bacillus subtilis, while the other is the production by the exogenous construct introduced in the genome under the Pveg constitutive promoter. This construction was introduced to counteract the leakiness of the promoter under XylR repression [BBa_K143036].

For AraR, which is produced only through the endogenous B. subtilis production, we considered the self-repression and described its production through a Hill function dependent on the concentration of the protein (Sá-Nogueira, 1997).

The final TF involved in our system is AraC, from E. coli, which is produced in our construct by the Pveg promoter. Its concentration depends exclusively on the maximal rate of transcription minus the general degradation rate. Since the repression method used by AraC is DNA looping, we used the same approach as in (Megerle J, 2011), where the repression intensity of AraC was defined in terms of arabinose instead of AraC concentration. In this case, the Hill coefficient used was n=3, as reported in the same source as the best fitting value. Under the same assumption of independence between the two TF involved in the hybrid promoter, the total repression intensity is the repression given by AraC DNA looping plus the intensity of XylR, both modeled with a Hill function.

Conclusions:

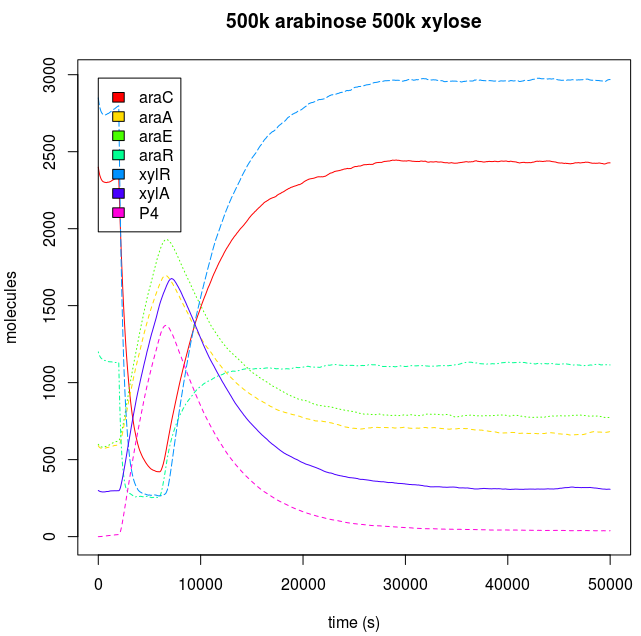
The complex mixture of the nature of the inputs used and their regulation of the designed system gives us a very interesting behavior. First, we want to highlight the existence of two unique steady states that are derived from the kind of addition of the inputs: one is reached when after an initial addition of sugars, these are degraded (in this case, there is an interval of time that shows the increment of the downstream protein and then its production rate decays as the sugars are degraded until they reach the state preceding the addition of sugars) and the other is when the amount of sugars is constant in the environment (like when using a chemostat the cell reaches a new state where there’s an equilibrium between the sugar catabolism and the continuous production of the downstream TF in the AND). The last remark is about the contribution to the AND by each input. We could see that when we had null or low levels of xylose, the repression remained strong. In the case of arabinose, its concentration contributes to the max level of expression of the downstream TF and has an impact on the way curve of expression behaves. Our hypothesis is that the relevance of arabinose is due to the fact that it plays a role in the regulation of the transportes, which has as its job the introduction of both kind of sugars. Our hypothesis is base on the fact that we see in the gradient graphs the decay of the transporter, followed by a sudden increase of it.